Section: New Results

A posteriori error analysis of sensitivities

Participants : Roland Becker, Daniela Capatina, Robert Luce, David Trujillo.

Most practical applications involve parameters $q={\left(q_i\right)}_{1\le i\le N}$ of different origins: physical (viscosity, heat conduction), modeling (computational domain, boundary conditions) and numerical (mesh, stabilization parameters, stopping criteria, values of a turbulence model). Numerical simulations can provide information related to the (first order) sensitivity of a quantity of physical interest $I\left(q\right)$ with respect to different parameters: $\partial I/\partial q_i$. Their computation can help to validate the physical model, to explain unexpected behaviour and also to guide efforts to improve both the physical and the computational models.

A posteriori error estimates for the functional itself, for fixed values of the parameters $q$, are well-known, cf. for example  [47] where a goal-oriented error control is achieved by introducing an adjoint problem. Our goal is to provide a general framework for the a posteriori error estimation of sensitivities $\partial I/\partial q_i-\partial I_h/\partial q_i$, which has not been given yet in the literature.

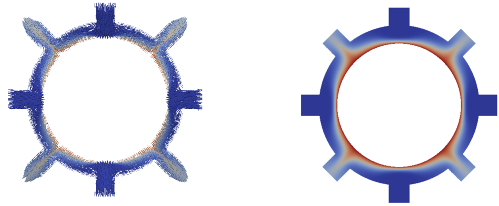
So far, we have applied the proposed method to the computation of the Nusselt number measuring the efficiency of a cooling process, described in the project Optimal. A cold liquid is injected in a annular domain through several inlets in order to cool a heated interior stator.

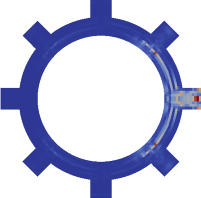
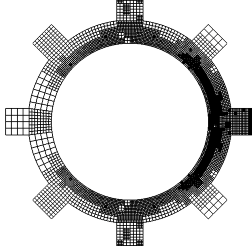
First numerical results, including adaptation with respect to the functional and to the sensitivity, have been carried out with the library Concha. They have been presented in [33] , [30] . In Figure 10 one may see the computed temperature and velocity field, while the a posteriori error estimator for the sensitivity of the Nusselt number with respect to the inflow speed at the right-hand side inlet, as well as the adapted mesh, are given in Figure 11 .

Figure 10. Computed velocity and temperature fields.

Figure 11. Error estimator for the sensitivity and adapted mesh.

In the future, several important aspects related to the adaptive method are still to be investigated such as design of an appropriate adaptive algorithm, proof of its convergence and optimality etc.